Ohm's Law
Combining
the elements of voltage, current, and resistance, Ohm developed the formula:
Where
·
V = Voltage in volts
·
I = Current in amps
·
R = Resistance in ohms
This is
called Ohm’s law. Let’s say, for example, that we have a circuit with the potential
of 1 volt, a current of 1 amp, and resistance of 1 ohm. Using Ohm’s Law we can
say:
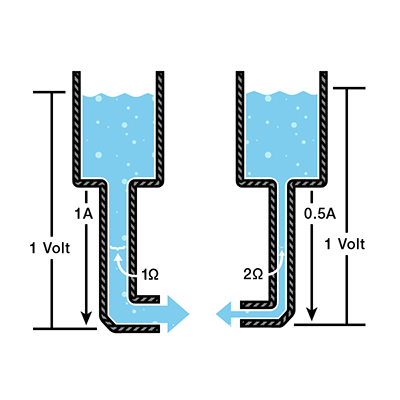
Let’s
say this represents our tank with a wide hose. The amount of water in the tank
is defined as 1 volt and the “narrowness” (resistance to flow) of the hose is
defined as 1 ohm. Using Ohms Law, this gives us a flow (current) of 1 amp.
Using
this analogy, let’s now look at the tank with the narrow hose. Because the hose
is narrower, its resistance to flow is higher. Let’s define this resistance as
2 ohms. The amount of water in the tank is the same as the other tank, so,
using Ohm’s Law, our equation for the tank with the narrow hose is
But
what is the current? Because the resistance is greater, and the voltage is the
same, this gives us a current value of 0.5 amps:
So, the
current is lower in the tank with higher resistance. Now we can see that if we
know two of the values for Ohm’s law, we can solve for the third.
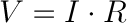
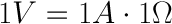
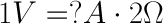
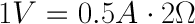
No comments:
Post a Comment